Science Seen Physicist and Time One author Colin Gillespie helps you understand your world.
The Point of the Universe
A new understanding of the universe is wending its way into our world. What is it? Where does it come from?
First let’s look at the old understanding. The Big Bang is the standard model of cosmology. It is based on general relativity and on evidence that space itself is expanding. It projects this picture back in time for 13.78 billion years and says the entire universe—space and the matter in it—started as a single point of zero size with infinite density and temperature. Is this the point of the universe? Well, actually hardly anybody buys the first picosecond of the model. Almost all say something different must have been there at the beginning. Whatever it was, it is by far the most interesting thing that ever was. What could it have been?
There is a simple answer. It sat on the sidelines for a century or more. Now it is coming into center view. In 1856, Bernhard Riemann mapped out a way to do geometry in a space that has multiple dimensions. It assumes space is continuous. Physics uses Riemannian geometry for Big-Bang cosmology to this day. Riemann noted another way that might be better: assume that space is made of little pieces that cannot be subdivided. He called them ‘elements of the manifold’. Let’s call them flecks. Their size is called the Planck size because Max Planck calculated it in 1899 when he invented quantum theory (for which he got a Nobel Prize). It is incredibly tiny. A fleck is to the period at the end of this sentence as that period is to the entire universe we see. Yet its non-zero size has deep meaning for future physics. For example, this is the realm string theory inhabits. Put Riemann and Planck and string theory together and you find the new point of the universe, the fleck, just one in the beginning. The image shows one view of such an object, magnified more than a million-billion-billion-billion times.
So, what if the universe began with a fleck instead of a point? If Riemann’s right and if space is made of 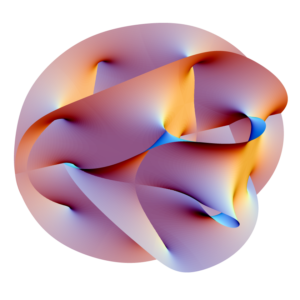 flecks there must now be many of them. The number needed to make the universe today would be more than two hundred digits long. How did there come to be so many? They must have replicated. The strange thing is that, starting with just one, their number needs to double fewer than a thousand times. This simple notion does away with all the contradictions of cosmological inflation.
flecks there must now be many of them. The number needed to make the universe today would be more than two hundred digits long. How did there come to be so many? They must have replicated. The strange thing is that, starting with just one, their number needs to double fewer than a thousand times. This simple notion does away with all the contradictions of cosmological inflation.
Now, what is all that stuff inside? Computer science professor Andrew Hansen figured how to make the image at the University of Indiana. It shows (as best a 2-D image can) the cooped-up six dimensions that string theory says the fleck should have. They’ll give rise to twists and tangles when it replicates. Working at the Perimeter Institute in Waterloo, Sundance Bilson-Thompson showed how twists and tangles like these could form all of the known sub-atomic particles. These particles have mass (or energy) and they make up the matter that we see.
On this view, the new point of the universe had no mass. Rather, mass emerges from the twists between flecks as the universe expands. And the extra dimensions that string theory needs are real. They were small from the beginning; no need to explain how they collapsed. They support the spooky action at a distance that physics calls entanglement, and explain dark energy, but that’s another post.
If the new point of the universe is real it calls for new ways of thinking. It should engage our interest because our world is made out of a gazillion copies and because it can explain so many puzzles.
How can the new point of the universe affect your life? Recall that old election slogan? It’s the economy! Think what we now do with quantum theory without any understanding of its meaning; and then imagine what we’ll do once we have physics that grasps what is really going on.
Sources:
Bernhard Riemann (1851), “Grundlagen für eine allgemeine Theorie der Functionen einer veränderlichen complexen Grösse”, Inauguraldissertation, Göttingen, http://www.emis.de/classics/Riemann/Grund.pdf; William Clifford (tr., 1873) “On the Hypotheses which lie at the Bases of Geometry”, Nature, vol. 8, p. 14 & p. 36; http://www.nature.com/nature/journal/v8/n183/pdf/008014a0.pdf & http://www.nature.com/nature/journal/v8/n184/pdf/008036a0.pdf
Other Reading:
Colin Gillespie (2013), Time One: Discover How the Universe Began, New York: RosettaBooks, p. 357, http://www.rosettabooks.com/book/time-one/; “Twist N Shout”, http://www.timeone.ca/chapters/twist-n-shout.pdf
Image credit: Andrew Hanson, https://www.cs.indiana.edu/~hanson/ and Wikimedia user Lunch, https://upload.wikimedia.org/wikipedia/commons/d/d4/Calabi-Yau.png

Blimey!