Reader Stevo asks a deep question: ‘If space is granular and Planck size is the shortest “length” then what geometric “shape” is a single Planck “thingie”?’

Geometry studies size and shape. Last week we saw Bernhard Riemann in 1856 divining how to do geometry in either of two different kinds of space: continuous space and granular space. Mainstream physics assumes space is continuous and uses Riemann’s math to do geometry, which leads to general relativity. But cutting-edge physics is turning toward granular space. String theories study the six-dimensional Calabi-Yau manifold. Its tiny scale, far smaller than an atom, was plumbed by Max Planck in 1899. Stevo wants to know about shape at that scale.

First let’s check how to find an answer in continuous space. We will require a metric. Think of it as a 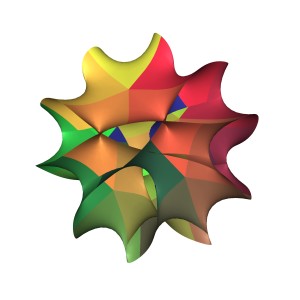ruler. It lets us measure distance and determine a straight line. Thing is, as Riemann pointed out, we can’t find a metric in continuous space. We have to invent one and bring it along. Now in a thought experiment we shrink ourselves and our metric down to Planck size and find: The curvature of space seems to be constant, otherwise the world looks just the same. That is: we can still define what shape is; but there are no Planck “thingies” to be found.

Now let’s shrink ourselves in Stevo-space. If we bring a metric with us it will be no use because it’s made of quanta of the space Stevo calls granular. Riemann calls them ‘elements of the manifold’, and I call them flecks. As Riemann pointed out 160 years ago we need no metric here. All that messy math of artificial metrics turns out to be fake. Just bring fingers and count flecks.

As we look around with gamma-plus-ray eyes what do we see? Not stacks of flecks like those depicted! 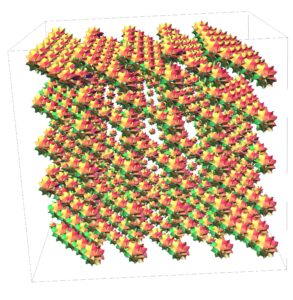Flecks don’t stack in space. They are space. Flecks and their next-neighbor relations are all that there is. So it’s not just that they do not stack; at Planck scale stacking has no meaning. To make matters worse for geometers, what we see changes every Planck time. If have our zeptoyoctoclock in hand we know that one tick is some fifty zeptoyoctoseconds. Not only does it all change every tick, we have no way to keep track of changes. If we move x flecks left or right, we can’t tell left from right or be sure that we did move or see where we moved from. In short it seems there is no concept of shape in the fleck landscape.

Now let’s get up close and friendly with a single fleck. Maybe that’s the shape that Stevo’s after. But we find it has no shape.  Not one of the computed shapes shape-master Andrew Hansen uses to illustrate a 6-D Calabi-Yau manifold on a 2-D page. Nor any other. Flecks have properties of volume, area, number, and next-neighbor relations. But no shape.

So what about those six internal dimensions? Don’t they have shape? Well no; what they have is topological relations, like twists and braids and knots. But there is no way—not even with a thought experiment—to climb inside a fleck and map its tangle. It has no inside. It just is.

But wait! When a fleck replicates, three 2-D pairs of internal dimensions link to its next neighbors. These links can have the property of twist. Twisted links can be braided. So here, Stevo, is what we see at Planck scale: No geometry, but topology. This is how Bilson-Thompson found the particles that make up the matter of the universe.

Sources:

Bernhard Riemann (1851), “Grundlagen für eine allgemeine Theorie der Functionen einer veränderlichen complexen Grösse”, Inauguraldissertation, Göttingen, http://www.emis.de/classics/Riemann/Grund.pdf; William Clifford (tr., 1873) “On the Hypotheses which lie at the Bases of Geometry”, Nature, vol. 8, p. 14 & p. 36; http://www.nature.com/nature/journal/v8/n183/pdf/008014a0.pdf & http://www.nature.com/nature/journal/v8/n184/pdf/008036a0.pdf

Other Materials:

Sundance Bilson-Thompson (2008), “A topological model of composite preons”, Cornell University Library, http://arxiv.org/abs/hep-ph/0503213

Colin Gillespie (2013), Time One: Discover How the Universe Began, New York: RosettaBooks, p. 349, http://www.rosettabooks.com/book/time-one/; “Making Space”, http://www.timeone.ca/chapters/making-space.pdf

Image credits: Andrew J. Hanson, https://www.cs.indiana.edu/~hanson/, https://en.wikipedia.org/wiki/Calabi%E2%80%93Yau_manifold#/media/File:CalabiYau5.jpg and https://upload.wikimedia.org/wikipedia/commons/a/a9/CY5-6D-lattice.jpg